This article delves into the multifaceted drivers influencing the EUR/CHF exchange rate across distinct economic periods. Our analysis meticulously identifies key breakpoints and subperiods, subsequently revealing the most pertinent factors shaping the EUR/CHF dynamics within each era. We further validate our findings through robustness checks, comparing our selected drivers with those derived from alternative methodologies and examining the real effective exchange rate.
Identifying Economic Regime Shifts: Breakpoints and Subperiods
To accurately analyze the EUR/CHF exchange rate, it’s crucial to identify shifts in economic regimes that may alter market behavior. We employ a statistical approach to pinpoint these breakpoints, which are then cross-referenced with significant economic events to ensure plausibility. Ideally, this method should accurately reflect the Swiss National Bank’s (SNB) minimum exchange rate policy timeline.
Our methodology involves constructing numerous regression models, each drawing at most one variable from predefined categories (e.g., stock indices, volatility indices) to mitigate multicollinearity. We then estimate breakpoints, limiting the maximum number to three and setting a minimum subperiod length of 20 months to ensure sufficient data for parameter estimation. Considering the timeline of events, we anticipate breakpoints coinciding with the Global Financial Crisis and the SNB’s peg period.
Table 1 below illustrates the frequency of breakpoint detections across 76 models that identified three breakpoints, using a minimum subperiod size of 20 months and a maximum of three breakpoints.
Table 1 Breakpoints Estimated with Minimal Subperiod Size of 20 Months and Maximum of Three Breakpoints
Alt Text: Table showing the frequency distribution of estimated breakpoints for EUR/CHF exchange rate analysis, clustered around the Global Financial Crisis and the Swiss National Bank’s minimum exchange rate policy period.
The analysis reveals breakpoint clusters around the Global Financial Crisis onset and the SNB’s minimum exchange rate policy’s beginning and end. Based on statistical findings and economic event timelines, we identify September 2011 and January 2015 as the most probable breakpoints for the SNB peg period. Given the use of monthly averages and the peg period’s start/end falling mid-month, we exclude these months from subperiods, potentially explaining why our statistical method detects adjacent months rather than the exact policy start/end.
For the initial breakpoint, several candidates emerge in the latter half of 2007. To resolve this, we select December 2007, which yields the highest overall adjusted R-squared (31.41%, adjusted 23.34%) when optimizing variables for each subperiod. Similar to the peg period, December 2007 is excluded from the first two subperiods.
In conclusion, our breakpoint analysis delineates the following subperiods for EUR/CHF exchange rate analysis:
- January 2000–November 2007: Pre-financial crisis
- January 2008–August 2011: Financial crisis to peg period onset
- October 2011–December 2014: SNB minimum exchange rate peg period
- February 2015–December 2020: Post-peg period
During the peg period, we also analyze a latent EUR/CHF exchange rate, derived from option pricing theory by Hanke et al. (2019), representing the exchange rate’s hypothetical trajectory absent the SNB’s intervention. Descriptive statistics for all variables across these subperiods are available in Table 9, and Figure 3 illustrates rolling window correlations between explanatory variables and the EUR/CHF exchange rate over an 18-month window.
Unpacking the Drivers of EUR/CHF Exchange Rate
For each identified subperiod, we employ a step-forward regression approach to pinpoint the most influential drivers of the EUR/CHF exchange rate. This method incrementally selects the best-performing subset of drivers from a pool of candidates, guided by Newey-West adjusted p-values. To mitigate multicollinearity risks associated with correlated drivers, we use Mallows’s Cp as a stopping criterion and monitor the Variance Inflation Factor (VIF) for each parameter.
In the regression tables that follow, all independent variables are standardized to facilitate interpretation, where coefficients represent the change in the dependent variable (EUR/CHF percentage changes, multiplied by 100) for a one-standard deviation increase in the driver.
Pre-Crisis Period Drivers (January 2000–November 2007)
Table 2 presents the regression results for the pre-financial crisis period (January 2000–November 2007). The DAX index emerges as the primary driver.
Table 2 OLS Regression Results: Pre-Crisis Period (January 2000–November 2007)
Alt Text: Regression analysis table displaying the impact of DAX and CHFUSD interest rate differential on EUR/CHF exchange rate during the pre-financial crisis period, highlighting DAX as a primary driver.
Column (1) indicates that before the crisis, a DAX decrease correlated with a stronger Swiss franc against the euro, aligning with the Swiss franc’s safe-haven asset status. The second selected driver is the CHFUSD 1-month interest rate differential (column 2). While CHFEUR differentials might seem more direct, CHFUSD differentials are preferred in this period, possibly due to a high correlation (53%) between CHFEUR1m and CHFUSD1m. The negative coefficient aligns with expectations: higher Swiss franc interest rates relative to the US dollar (and euro) strengthen the franc against the euro.
Model (2) in Table 2 exhibits the lowest Mallows’s Cp and is therefore selected as the optimal model for this period. While SMI emerges as a potential third driver, its inclusion (column 3) increases Mallows’s Cp, suggesting it’s not a significant addition. DAX and SMI are highly correlated, and including SMI results in coefficient instability and higher VIFs, despite a slight increase in adjusted R-squared. The overall explanatory power in this period is low, with an adjusted R-squared of only 3.9%.
Financial Crisis Period Drivers (January 2008–August 2011)
During the financial crisis period (January 2008–August 2011), the CHFUSD 10y2y yield curve slope difference is identified as the most significant driver of the EUR/CHF exchange rate (Table 3).
Table 3 OLS Regression Results: Financial Crisis Period (January 2008–August 2011)
Alt Text: Regression table showing CHFUSD yield curve slope difference, VSMI, and DAX as drivers of EUR/CHF exchange rate during the financial crisis, emphasizing the yield curve slope as the most significant factor.
Model (1) in Table 3 reveals that an increase in Switzerland’s 10y2y spread relative to the USD strengthens the Swiss franc against the euro. Adding VSMI and DAX further improves the model fit based on Mallows’s Cp. VSMI, as a crisis indicator, carries a positive coefficient, reinforcing the Swiss franc’s safe-haven status: increased equity volatility strengthens the franc.
The inclusion of DAX in model (3) changes its coefficient sign to negative, which might seem counterintuitive. However, this regime-dependent relationship between DAX and EUR/CHF (USD/CHF) is documented in prior research. This sign change and the increased VSMI coefficient magnitude in model (3) may stem from the correlation between DAX and VSMI. While Mallows’s Cp suggests DAX inclusion, CHFEUR10y2y is not recommended as the next variable due to compensatory effects with CHFUSD10y2y and increased VIF. The explanatory power significantly increases in this period to an adjusted R-squared of 20%.
Peg Period Drivers (October 2011–December 2014)
For the SNB peg period (October 2011–December 2014), the short-term interest rate differential between the Swiss franc and the euro (CHFEUR1m) is the sole significant driver of the EUR/CHF exchange rate (Table 4).
Table 4 OLS Regression Results: Peg Period (October 2011–December 2014)
Alt Text: Regression analysis table highlighting CHFEUR 1-month interest rate differential as the primary, and almost sole, driver of EUR/CHF exchange rate during the Swiss National Bank’s peg period.
Column (1) in Table 4 shows the expected negative relationship: higher Swiss franc interest rates strengthen it against the euro. Adding the term structure slope difference (column 2) increases R-squared but also Mallows’s Cp, and its positive coefficient is not statistically significant and alters the CHFEUR1m coefficient.
Columns (3) and (4) explore the impact of including VSMI as the first variable, as some correlation-based selection methods suggest. While VSMI inclusion improves R-squared, its coefficient is insignificant. CHFEUR1m then becomes the second driver with similar coefficients and p-values as in model (2). This illustrates the difference between p-value based (our approach) and correlation-based variable selection methods: the latter often achieve higher R-squared but at the cost of potentially insignificant coefficients.
Interestingly, Foreign Currency Investments (FCI) are not selected as a driver during the peg period. This could be attributed to the SNB’s strong commitment to the lower bound of 1.20, requiring less FCI intervention except during periods of market stress. Figure 1 indicates FCI spikes during intervention periods in Q3 2011 and Q2 2012, followed by relative stability. However, we find no significant FCI influence during the peg period.
The explanatory power in the peg period is very low, with an adjusted R-squared of only 1.6%, possibly due to the SNB’s dominant policy influence. Table 5 examines the peg period using the latent EUR/CHF exchange rate.
Table 5 OLS Regression Results: Peg Period with Latent Exchange Rate (October 2011–December 2014)
Alt Text: Regression table analyzing drivers of the latent EUR/CHF exchange rate during the peg period, showing CHFEUR 1-month interest rate differential and DAX as significant drivers when SNB intervention effects are removed.
Using the latent exchange rate, CHFEUR1m and DAX become significant drivers (Table 5). The signs are consistent with previous interpretations. Comparing CHFEUR1m coefficients in Tables 4 and 5 reveals that the SNB’s peg policy significantly dampened the impact of short-term interest rate differentials on the observed EUR/CHF exchange rate.
Post-Peg Period Drivers (February 2015–December 2020)
The post-peg period (February 2015–December 2020) exhibits the highest number of significant EUR/CHF drivers. FCI emerges as a significant driver for the first time, with high significance (Table 6).
Table 6 OLS Regression Results: Post-Peg Period (February 2015–December 2020)
Alt Text: Regression table highlighting Foreign Currency Investments (FCI), SMI, and CHFEUR 30-year interest rate differential as key drivers of EUR/CHF exchange rate in the post-peg period.
The positive FCI coefficient indicates that increased FCI led to Swiss franc weakening, as expected. The SMI is the second selected variable, with a positive coefficient, suggesting portfolio channel demand for Swiss assets strengthens the franc. Adding the long-term Swiss franc–euro interest rate differential (CHFEUR30y) further improves the model (column 3), with the expected negative sign. Including the EURUSD1m interest rate differential does not further improve Mallows’s Cp. Model (3), containing FCI, SMI, and CHFEUR30y, is selected for its sparsity among models with significant coefficients, achieving a high adjusted R-squared of 23.6%. Even model (1) with only FCI has an adjusted R-squared of 16.7%.
Summary of Regression Findings
Table 7 summarizes the best models for the EUR/CHF exchange rate across all subperiods.
Table 7 Summary of Best Model OLS Regression Results Across Subperiods
Alt Text: Summary table comparing the best regression models for EUR/CHF exchange rate drivers across pre-crisis, financial crisis, peg period, and post-peg economic subperiods.
Larger coefficients (absolute terms) indicate a stronger driver impact due to variable standardization. The largest coefficients and highest R-squared values are observed during the crisis and post-peg periods. The pre-crisis and peg periods exhibit lower driver impact and explanatory power.
Our analysis reveals varying drivers across categories and subperiods, with some drivers even changing sign across regimes. These findings align with existing literature documenting time-varying relationships between exchange rates and macroeconomic factors. Our descriptive approach identifies these shifts without explicitly modeling the underlying economic mechanisms. Further research is needed to explore why driver significance and impact vary across subperiods.
Robustness Checks: REER Drivers and Alternative Variable Selection
To validate our methodology, we conducted robustness checks by repeating the analysis using the Swiss real effective exchange rate (REER) and comparing drivers to those found for the observed EUR/CHF rate. We also compared our driver selection to alternative methods.
Table 10 in the Appendix presents the best models for the REER, and Table 8 compares REER drivers to EUR/CHF drivers and those selected by alternative variable selection procedures.
Table 8 Driver Comparison Across Subperiods and Selection Methods
Alt Text: Comparative table showing drivers of EUR/CHF and Real Effective Exchange Rate (REER) across different economic periods, contrasted with drivers selected by alternative variable selection methods.
Table 8 shows considerable overlap in drivers between REER and EUR/CHF (approximately half are identical). The changes in beta coefficient signs in Table 10 (Appendix) compared to Table 7 are due to REER calculation conventions: Swiss franc appreciation strengthens REER but decreases the EUR/CHF rate.
Comparing our p-value-based approach to alternatives reveals broad agreement on the initial drivers across regimes, except for the peg period. The main difference is our method’s tendency towards sparser models. In the peg period, some alternatives select VSMI as the primary driver, whereas our approach favors CHFEUR1m, as discussed in section 4.2.3.
Figure 3 Rolling Window Correlations between Explanatory Variables and EUR/CHF Exchange Rate
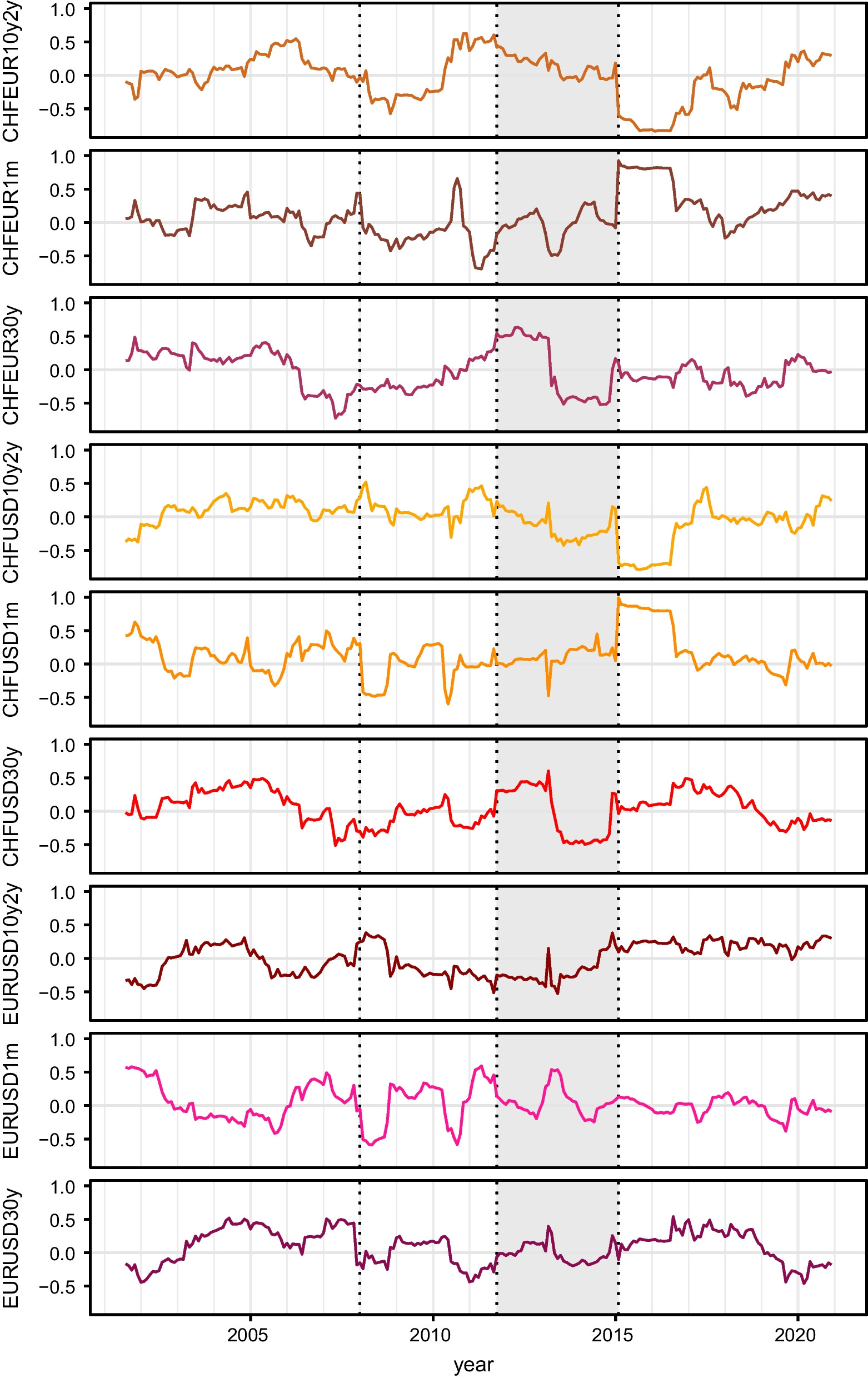Figure 3: Rolling Window Correlations EUR/CHF Drivers
Alt Text: Line graph illustrating rolling window correlations over 18 months between various explanatory variables and the EUR/CHF exchange rate, showing the dynamic relationships over time.
Appendix Table 10 OLS Regression Results for Real Effective Exchange Rate (REER)
Alt Text: Appendix table presenting detailed OLS regression results for the Swiss Real Effective Exchange Rate (REER) across different economic periods, used for robustness checks against EUR/CHF driver analysis.
